Exploring Acute Angles: Definition, Examples, and Properties
Comprehensive Definition, Description, Examples & Rules
Discover the Mind-Blowing Secrets of Acute Angles! With Edulyte as your ultimate learning companion, say goodbye to confusing geometry. With our free resource, you can explore the acute angle meaning and an acute angle example and the properties of an acute angle and its practical applications like a geometry wizard!
Introduction to Acute Angles
Acute angles are fundamental in geometry, offering a unique perspective on shapes and spatial relationships. Understanding what acute angles are and their significance is essential for learners of English as well as parents supporting their children’s mathematical education.
Definition and Concept of Acute Angles
How do you define acute angle? An acute angle is a specific type of angle that measures less than 90 degrees. In simpler terms, they are angles that are smaller than a right angle. An acute angle degree will always be less than 90 degrees. Imagine a pizza slice smaller than a quarter of the whole pizza – that’s an acute angle! Acute angles are characterised by their sharpness and how they “squeeze” space. They are like the building blocks of angles, forming the basis for other angle measurements.
Understanding acute angles is crucial because they provide a foundation for comprehending more complex geometric concepts. By grasping the idea of acute angles, you can confidently and accurately navigate the vast world of geometry.
Real-Life Applications of Acute Angles
The measure of acute angle is always less than 90 degrees. Acute angles have practical applications in various real-life scenarios. Let’s consider a few examples:
- Architecture: Architects use acute angles when designing structures. They consider the angles formed by the intersection of walls, windows, and roofs to create aesthetically pleasing and structurally sound buildings.
- Engineering: Engineers design bridges, roadways, and machinery using acute angles. Understanding how acute angles affect the stability and functionality of structures is crucial for engineering success.
- Navigation: Navigators use acute angles to determine the direction of their vessels. They can plot accurate courses by interpreting angles formed between landmarks or celestial bodies.
Meaning and Measurement of Acute Angles
Let’s explore their meaning and measurement to understand acute angles better.
Acute angles, as mentioned earlier, are angles that are less than 90 degrees. An acute angle example can include any angle from a small angle close to zero degrees to an angle shy of a right angle, which measures 89 degrees. Imagine holding a protractor against a straight line, and the acute angle would be the opening between the two sides of the protractor.
In geometry, angles are often measured in degrees, representing a fraction of a circle. A full circle contains 360 degrees, and an acute angle measures less than one-fourth of that circle.
Understanding the measurement of acute angles is not limited to degrees alone. In some cases, angles can be measured in radians or other units of angular measurement. For example, radians are commonly used in trigonometry to measure angles in equations and calculations. It is helpful for you to grasp the concept of converting between degrees and other angular units, expanding your mathematical fluency.
Examples of Acute Angles
You can spot an acute angle in various objects and scenarios around us. Let’s explore some everyday examples:
- A slice of pizza: Picture a triangular slice of pizza. The angle at the tip of the slice is acute, as it is smaller than 90 degrees.
- Roof angles: The angles formed by rooflines are often acute in architecture. The steepness of the roof pitch relies on acute angles to efficiently shed rainwater and snow.
- Road signs: Traffic signs, such as yield signs or sharp turn warnings, often feature acute angles to indicate the direction or severity of a curve.
Types of Angles in Geometry
Acute angles are just one type of angle in geometry. It is helpful to have a broader understanding of different types of angles in geometry, their angle measures and their relationships. Here are a few key types of angles:
- Right angles: These angles measure precisely 90 degrees and form a perfect L-shape. They are found at the corners of squares, rectangles, and other perpendicular intersections.
- Obtuse angles: Unlike acute angles, obtuse angles are larger than 90 degrees but smaller than 180 degrees. They have a wider opening and can be visualised as an open-angle, like a reclining chair.
- Straight angles: Straight angles are a crucial type of angles in geometry, measuring exactly 180 degrees and forming a straight line. They can be visualised as the fusion of two right angles.
- Reflex angles: Moving beyond the straight angle, reflex angles range between 180 and 360 degrees, offering a more extensive sweep around a circle’s edge.
- Complementary angles: A pair of angles that together add up to 90 degrees. This type of angles in geometry showcases how two angles can pair up to achieve the right angle.
- Supplementary angles: These angles sum up to 180 degrees, forming a straight angle when combined.
- Adjacent angles: Sitting side by side, adjacent angles share a common vertex and side, but not any interior points.
- Vertical (or opposite) angles: Upon the intersection of two lines, they create two pairs of equal-measured vertical angles.
As we explore the type of angles in geometry, it becomes clear how varied and complex these angular relationships can be, paving the way for more complex geometric concepts.
Properties of Acute Angles
Acute angles possess specific properties that contribute to their significance in geometry. Let’s explore some of these properties:
- Complementary angles: When two acute angles add up to precisely 90 degrees, they are called complementary angles. For example, an angle measuring 40 degrees and another measuring 50 degrees are complementary.
- Supplementary angles: Acute angles can also be supplementary, meaning they add up to 180 degrees when combined with another angle.
Tip: Acute angles cannot be supplementary to each other, as their sum would exceed 180 degrees.
Understanding the properties of acute angles allows learners to identify relationships between angles and solve geometric problems more effectively.
Importance of Acute Angles
Acute angles have far-reaching implications in various fields and everyday life. Here are some key reasons why understanding acute angles is essential:
- Architecture and Design: Architects use acute angles to create visually appealing and structurally sound buildings. Using sharp angles improves architectural designs’ stability, aesthetics, and functionality.
- Engineering and Construction: Engineers utilise acute angles in designing bridges, roadways, and machinery. Understanding the impact of acute angles on load distribution and structural integrity is vital for engineering projects.
- Critical Thinking and Spatial Reasoning: Comprehending and working with acute angles develops critical thinking skills and spatial reasoning abilities.
By grasping the importance of acute angles in these fields, learners can appreciate their practical applications and the value of geometry.
Step Up Your Math Game Today!
Free sign-up for a personalised dashboard, learning tools, and unlimited possibilities!

Key Takeaways
- Definition: An acute angle is an angle that measures less than 90 degrees..
- Measurement: Acute angles range from 0 degrees to just under 90 degrees.
- Complementary Angles: Acute angles can form pairs of complementary angles when their measures add up to 90 degrees.
- Trigonometry: Trigonometric functions such as sine, cosine, and tangent can be defined and calculated for acute angles.
- Geometric Properties: Acute angles help determine the shape and properties of various geometric figures.
- Applications: Acute angles are found in many real-life scenarios, such as roof slopes, road intersections, and triangular signs.
Quiz
Question comes here
Frequently Asked Questions
No, an acute angle cannot be greater than 90 degrees. By definition, an acute angle is any angle that measures less than 90 degrees. It is considered a right angle if an angle measures precisely 90 degrees. Angles that measure greater than 90 degrees are called obtuse angles.
There are numerous real-life examples of acute angles. Here are a few:
- Roof Slopes: The slope of a pitched roof often forms acute angles at the edges. These angles help shed water and prevent it from accumulating on the roof.
- Intersection of Roads: At intersections, the angles formed by the crossing roads are often acute. They determine the direction of traffic flow and aid in smooth navigation.
- Triangular Signs: Many road signs, such as yield or warning signs, have triangular shapes with acute angles. These angles contribute to the distinctive form and visibility of the signs.
- Mountain Peaks: When viewing a mountain range, the peaks typically form acute angles with the ground. These angles contribute to the steepness and ruggedness of the terrain.
- Converging Lines: In architectural designs, converging lines, such as those in the corner of a building or the edges of a picture frame, often form acute angles. These angles add structure and aesthetics to the overall design.
- Place the centre point of the protractor at the vertex (the point where the two lines of the angle meet).
- Align the baseline of the protractor (the straight edge at the bottom) with one of the angel’s arms (one of the lines extending from the vertex).
- Read the measurement where the other arm of the angle intersects with the protractor scale. Use the appropriate scale (usually labelled in degrees) and note the value where the line crosses it.
- The value you read on the protractor measures the acute angle in degrees.
To identify acute angles in geometric figures, you can follow these steps:
- Understand the definition: Acute angles measure less than 90 degrees. Keeping this definition in mind will help you identify acute angles accurately.
- Examine the angle’s measurement: If you have access to it, check if it falls within the 0 to 90 degrees range. If the angle measures less than 90 degrees, it is acute.
- Compare with a right angle: Compare the angle in question to a right angle, which measures precisely 90 degrees. If the angle appears smaller or narrower than the right angle, it is acute.
- Analyse the figure’s properties: Look at the geometric figure. If you notice angles that appear less than 90 degrees, they are likely acute angles. Pay attention to angles formed by intersecting lines, corners of polygons, or sides of triangles.
The properties of acute angles include the following:
- Measurement: Acute angles always have a measurement of less than 90 degrees. They can range from a fraction of a degree up to just under 90 degrees.
- Shape: Acute angles are characterised by their sharpness and narrowness. They appear smaller and narrower than a right angle (90 degrees) or an obtuse angle (greater than 90 degrees).
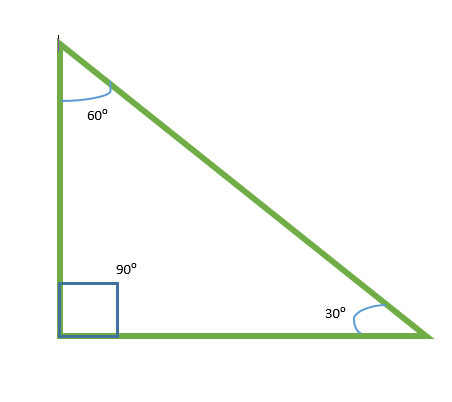
- Triangle Classification: In a triangle, if all three angles are acute, the triangle is classified as an acute triangle. An acute triangle has all interior angles measuring less than 90 degrees.
- Complementary Angles: Acute angles can form complementary angles when combined with another angle. Complementary angles add up to 90 degrees. For example, an acute angle of 30 degrees and a complementary angle of 60 degrees form a pair of complementary angles.
- Trigonometric Functions: Trigonometric functions such as sine, cosine, and tangent can be defined and calculated for acute angles. These functions are used extensively in trigonometry and have specific properties and formulas applicable to acute angles.
Understanding acute angles is essential for solving various geometric problems. Here are a few ways in which understanding acute angles can be beneficial:
- Angle Relationships: Acute angles are crucial in understanding angle relationships within geometric figures. They help identify complementary angles (angles that add up to 90 degrees) and supplementary angles (that add up to 180 degrees), allowing you to solve equations involving these angle measures.
- Triangle Properties: Acute angles are present in acute triangles, with all three interior angles measuring less than 90 degrees. Understanding acute angles helps analyse and solve problems related to acute triangles’ sides, angles, and properties.
- Trigonometry: Trigonometric functions such as sine, cosine, and tangent are commonly used to solve problems involving acute angles in trigonometry. These functions help calculate side lengths, angle measures and solve real-world problems involving triangles and angles.
- Construction and Measurement: When constructing geometric figures or measuring angles, understanding acute angles allows for accurate placement and alignment. It helps create precise drawings and measurements of acute angles within geometric constructions.
- Problem-Solving: Many geometric problems and proofs involve working with acute angles. Understanding their properties and relationships enables you to apply geometric principles and solve problems effectively.